South Australian Medical Heritage Society Inc

Plethysmographs

ACKNOWLEDGEMENT
We are grateful to Prof. Alan Crockett for allowing us to use photographs of some of the plethysmographs from his lab, and providing further information; and to Allen Gale? for the BodyTest plethysmograph photograph.

A plethysmograph is a device which measures the changes in the volume of an object. The most common use is to calculate lung volume and airway resistance by the measurement of pressures and an application of Boyle's law. The word "plethysmograph" is derived from the Greek plēthusmos ("enlargement") and graphein ("to write"), and was coined some time around 1870-75.

There are two main classes of body plethysmographs, the constant volume (Dubois), and the variable volume (Mead). Both types can be used to determine various lung volumes (functional residual capacity, aka FRC; maximum, tidal volume, etc), flow rates, the airway resistance, and diagnose restrictive and obstructive diseases.
¿Variable volume boxes can also be used in gas compression studies and lung compliance (the ease of extension) measurements. ? gas compression studies? what for? just exhalation force/volume?

Fick's volumzeichner ("volume drawer").

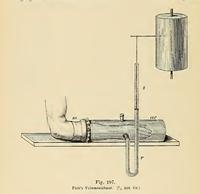
The picture above is an example of a plethysmograph recording the changes in volume of a forearm, taken from Physiologische Graphik: Ein leitfaden der in der physiologie gebräuchlichen registrirmethoden "Physiological graphics: A guide to the methods of registration commonly used in physiology"), Dr. Oskar Langendorff, 1891, pg 236. The container is filled with water, as is the U-tube. As the arm changes in volume, the float in the tube rises and falls, and its height is recorded on a rotating drum.

One of the earliest recorded body plethysmographs was made by the Dutch biologist Jan Swammerdam in 1667. In an effort to prove his mistaken idea that the expanding chest pushed air into the lungs, Swammerdam placed a tube into the throat of a dog, and then submerged it in a barrel of water. As the dog breathed in and out, the water level rose and fell.

A similar experiment was conducted by the Scottish Dr. Robert Menzies in his thesis, "A dissertation on respiration" in 1790. He repeatedly blew into a bag of a known volume (2,400 cubic inches, or 39.33 L) until it was full (~56 breaths), averaging 42.8 cubic inches (701.4 mL) per breath.

He checked this by placing a person in a barrel of water up to their neck, and recorded the changing height of the water as they breathed. From this he could calculate the changes in volume as a result of breathing. He found that the bag method and water immersion method both yielded the results.

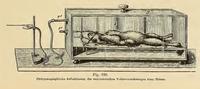
These two diagrams show examples of early plethysmographs. The first picture is of a sealed box containing a rabbit. There is a short tube running from outside the box into the rabbits trachea, allowing it to breath. The longer tube leads to an external pressure recorder, in this case a Marey-style recording needle, which would then scratch the recording onto a sheet of lamp black (carbon black) covered paper on a rotating drum (not shown in this picture), much like the phonautograph did with sound.
The other picture is of the type of plethysmograph used by J. S. Haldane and J. G. Priestley to record the amount of air breathed in their research into how CO₂ levels affected breathing. The the lower tube allows for calibration and venting of the box, and the other tube leads to a pressure recorder.

A modern Mead variable volume plethysmograph.

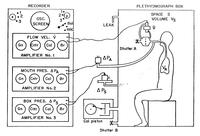
The picture above is a more recent example of a variable volume plethysmograph. This type of box was designed in 1960 by Dr Jere Mead, and is similar to the box used by Haldane and Priestley.
The subject breathed air from outside of the box. The resulting change in box volume was recorded by a spirometer or calculated from a pneumotachograph. The pressure at the mouth could be measured directly, and the amount of air breathed was recorded by a pneumotachograph.

A schematic of Dubois' early design.
add source?

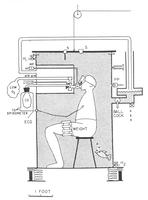
The picture above shows a schematic design of one of Dubois' early plethysmograph designs. The person sat inside a large box filled with warm water, which would allow for easy monitoring of volume changes. It is reported that as the person left wouldn't entering be more problematic? the box, it flooded the Dean's office below. For some strange reason, this design did not last long.

In 1955, Dr Arthur DuBois, et al, described a new way to calculate the amount of air in a person. This method was based on Eduard Pfluger's 1882 idea of the voluntary compression and decompression of gas in the lungs. It was faster, easier to perform, and more accurate than the existing techniques.

A schematic of DuBois' plethysmographic setup.
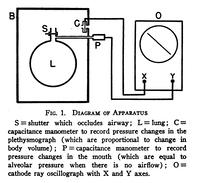
From: The Journal of Clinical Investigation, vol 35, issue 3, pg 322-326

The subject sat inside a sealed box of approximately 600L, roughly the size of a telephone booth, until the temperature inside had stabilized, and the pressure inside the box was adjusted to be equal to the external atmospheric pressure.
The subject would then breathe through a mouthpiece that could be closed remotely. At the end of a normal exhalation (when the lungs volume is the functional residual capacity, or FRC), a shutter inside the mouthpiece closed, and the subject would then rapidly try to breath in and out (~2Hz) a few times, then the shutter would be opened again. The pressure at the mouth (which is the approximatly the lung pressure when breathing freely), and inside the box were recorded simultaneously. From this, and the volume of the box, one could find the volume of air in the subject using Boyle's law, which states that for a constant temperature, pressure time volume is constant.

So, if we call the thoracic pressure at the end of exhalation P₁, which is the same as the box pressure (otherwise, they would still be exhaling), the it follow that the pressure at the end of the attempted inhalation of P₂ = (P₁ + ΔP), where ΔP is the difference in mouth pressure before and after. Similarly, the thoracic volumes are V₁ after exhalation, and V₂ = (V₁ + ΔV) after inhalation.

P₁V₁ = P₂V₂
P₁V₁ = (P₁ + ΔP)(V₁ + ΔV)
P₁V₁ = P₁V₁ + P₁ΔV + ΔPV₁ + ΔPΔV
0 = P₁ΔV + V₁ΔP + ΔPΔV
-V₁ΔP = P₁ΔV + ΔPΔV
V₁ΔP = -ΔV(P₁ + ΔP)
V₁ = -ΔV(P₁ + ΔP) / ΔP

As ΔP is much less than the atmospheric pressure, this approaches:

V₁ = -ΔVP₁ / ΔP     (eq 1)

Since negative volume makes no sense, we can write:

V₁ = P₁(ΔV / ΔP)

As we know that the pressure at the mouth (P₁is very nearly at atmospheric pressure (K_v), that in a closed system the change in lung volume must be the equal and opposite to the change in the box volume, and that V₁ is the thoracic gas volume (also know as the Functional Reserve Capacity), we can rewrite eq. 1 as:

FRC = K_v(ΔV_box / ΔP_mouth)
why is it called K_v?

In increased FRC what constitutes increased? absolute volume? standard deviations for height/weight? can be indicative of an obstructive disease such as asthma or emphysema, while a decreased FRC may indicate a reduced compliance (elastisity), and a restrictive disease.

The airway resistance can then be calculated from the difference in airway pressure and the flow rate of the air (the derivative of volume with respect to time):

R = ΔP_mouth / (dV/dt)

One might note the similarity to Ohm's law, Resistance = Voltage / Current. As current (I) is the time derivative of charge (dQ/dt), this can be written as R = V / (dQ/dt).

The airway resistance could also be calculated graphically. By plotting the flow rate, dV/dt on the y-axis; and ΔP on the x-axis of an oscilliscope, the resistance equals

R = K_p/K_f . x / y_f . cot(p)

A schematic of Dubois' plethysmograph and analyzer from around 1955.
check date, add source?

check
The oscilliscope was used to plot two variables. When plotting the box pressure versus the pressure at the mouth, the operator was able to calculate the volume of air in the subject. Alternatively, by plotting the change in mouth pressure versus the flow rate, one could determine the airway resistance from the slope of the trace.

The following photographs were kindly provided by Prof. Alan Crockett.

A Jäger BodyTest unit (left) with control machine (right). A Dubois type full body constant volume plethysmograph from c. 1978.